What Can Be Used to Determine the Relative Age of Two Rocks

DETERMINING AGE
OF ROCKS AND FOSSILS

FRANK Thousand. MCKINNEY

THE AGE of fossils intrigues nigh everyone. Students non merely want to know how old a fossil is, but they want to know how that historic period was determined. Some very straightforward principles are used to decide the historic period of fossils. Students should be able to understand the principles and have that as a background so that age determinations by paleontologists and geologists don't seem like black magic.

At that place are two types of age determinations. Geologists in the late 18th and early 19th century studied stone layers and the fossils in them to determine relative age. William Smith was one of the nigh of import scientists from this fourth dimension who helped to develop knowledge of the succession of different fossils by studying their distribution through the sequence of sedimentary rocks in southern England. It wasn't until well into the 20th century that enough data had accumulated about the rate of radioactivity that the historic period of rocks and fossils in number of years could be determined through radiometric age dating.

This activity on determining historic period of rocks and fossils is intended for 8th or ninth course students. It is estimated to require 4 hours of grade fourth dimension, including approximately i hour total of occasional educational activity and explanation from the instructor and two hours of group (team) and individual activities by the students, plus one hour of discussion among students within the working groups.

Explore this link for additional data on the topics covered in this lesson:

• Geologic Fourth dimension

PURPOSE AND OBJECTIVES

This activity will help students to have a better agreement of the basic principles used to determine the age of rocks and fossils. This action consists of several parts. Objectives of this activity are:
1) To accept students determine relative age of a geologically complex expanse.
2) To familiarize students with the concept of half-life in radioactivity.
3) To take students see that individual runs of statistical processes are less predictable than the average of many runs (or that runs with relatively small-scale numbers involved are less dependable than runs with many numbers).
iv) To demonstrate how the rate of radioactive decay and the buildup of the resulting disuse product is used in radiometric dating of rocks.
5) To use radiometric dating and the principles of determining relative age to show how ages of rocks and fossils tin exist narrowed even if they cannot be dated radiometrically.
Return to summit

MATERIALS REQUIRED FOR EACH GROUP

one) Block diagram (Effigy 1).
ii) Large cup or other container in which Chiliad & M'due south can be shaken.
3) 100 M & Thousand'south
4) Graph paper (Figure 2).
five) Watch or clock that keeps fourth dimension to seconds. (A unmarried watch or clock for the entire class volition exercise.)
vi) Piece of paper marked TIME and indicating either 2, 4, half-dozen, eight, or 10 minutes.
7) 128 small cards or buttons that may be cut from cardboard or construction paper, preferably with a different color on opposite sides, each marked with "U-235" all on one colored side and "Lead-207" on the reverse side that has some contrasting colour.

 

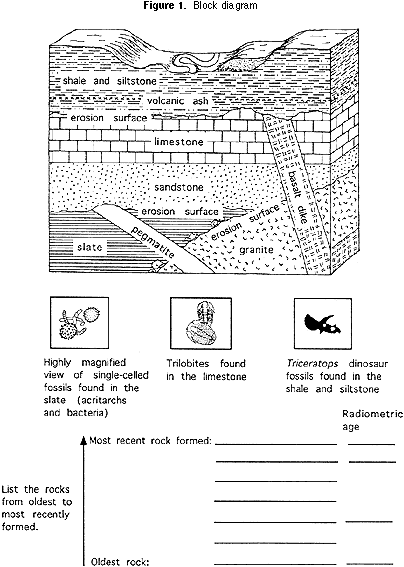
Return to top

Office i: DETERMINING RELATIVE Age OF ROCKS

Each team of three to 5 students should hash out together how to decide the relative historic period of each of the rock units in the block diagram (Effigy 1). After students accept decided how to plant the relative age of each stone unit of measurement, they should list them under the block, from well-nigh recent at the top of the listing to oldest at the lesser.

The teacher should tell the students that there are two basic principles used by geologists to determine the sequence of ages of rocks. They are:
Principle of superposition: Younger sedimentary rocks are deposited on top of older sedimentary rocks.
Principle of cross-cut relations: Any geologic feature is younger than anything else that information technology cuts across.

PART 2: RADIOMETRIC AGE-DATING

Some elements have forms (called isotopes) with unstable atomic nuclei that have a tendency to change, or decay. For case, U-235 is an unstable isotope of uranium that has 92 protons and 143 neutrons in the nucl eus of each atom. Through a serial of changes within the nucleus, it emits several particles, ending up with 82 protons and 125 neutrons. This is a stable status, and in that location are no more changes in the atomic nucleus. A nucleus with that number of protons is chosen lead (chemical symbol Lead). The protons (82) and neutrons (125) total 207. This particular form (isotope) of lead is called Lead-207. U-235 is the parent isotope of Pb-207, which is the daughter isotope.

Many rocks contain small amounts of unstable isotopes and the daughter isotopes into which they disuse. Where the amounts of parent and daughter isotopes can be accurately measured, the ratio can exist used to determine how old the rock is, equally shown in the following activities.

Part 2a Activity — At whatsoever moment there is a modest gamble that each of the nuclei of U-235 will suddenly decay. That chance of decay is very small-scale, but it is always present and it never changes. In other words, the nuclei do non "wear out" or get "tired". If the nucleus has non nevertheless decayed, there is e'er that same, slight adventure that it will change in the near hereafter.

Diminutive nuclei are held together by an attraction between the large nuclear particles (protons and neutrons) that is known as the "strong nuclear strength", which must exceed the electrostatic repulsion between the protons within the nucleus. In general, with the exception of the single proton that constitutes the nucleus of the most abundant isotope of hydrogen, the number of neutrons must at least equal the number of protons in an diminutive nucleus, because electrostatic repulsion prohibits denser packing of protons. Simply if in that location are likewise many neutrons, the nucleus is potentially unstable and decay may be triggered. This happens at any fourth dimension when addition of the fleeting "weak nuclear strength" to the ever-present electrostatic repulsion exceeds the binding energy required to hold the nucleus together.

Very careful measurements in laboratories, made on VERY LARGE numbers of U-235 atoms, have shown that each of the atoms has a l:fifty take a chance of decaying during about 704,000,000 years. In other words, during 704 million years, half the U-235 atoms that existed at the beginning of that time will decay to Pb-207. This is known as the half life of U- 235. Many elements take some isotopes that are unstable, essentially because they have too many neutrons to be balanced by the number of protons in the nucleus. Each of these unstable isotopes has its own feature half life. Some half lives are several billion years long, and others are as short as a ten-thousandth of a second.
Return to top

A tasty way for students to sympathise about one-half life is to give each team 100 pieces of "regular" Thou & M candy. On a piece of notebook paper, each piece should be placed with the printed M facing down. This represents the parent isotope. The processed should be poured into a container large enough for them to bounce around freely, it should exist shaken thoroughly, then poured back onto the newspaper and then that it is spread out instead of making a pile. This first time of shaking represents 1 half life, and all those pieces of candy that have the printed M facing upwards correspond a change to the girl isotope. The team should pick up and prepare aside But those pieces of candy that accept the M facing upwardly. So, count the number of pieces of processed left with the Thou facing downward. These are the parent isotope that did non change during the get-go half life.

The instructor should take each team report how many pieces of parent isotope remain, and the offset row of the decay table (Figure 2) should be filled in and the average number calculated. The same process of shaking, counting the "survivors", and filling in the side by side row on the decay table should be done vii or eight more than times. Each time represents a half life.

Later on the results of the concluding "one-half life" of the M& M are nerveless, the candies are no longer needed.

Each team should plot on a graph (Effigy 3) the number of pieces of processed remaining later each of their "shakes" and connect each successive signal on the graph with a light line. On the aforementioned graph each team should plot the AVERAGE VALUES for the class as a whole and connect that by a heavier line. AND, on the same graph, each group should plot points where, after each "shake" the starting number is divided past exactly two and connect these points by a differently colored line. (This line begins at 100; the next point is 100/ ii, or 50; the next indicate is 50/2, or 25; and so on.)

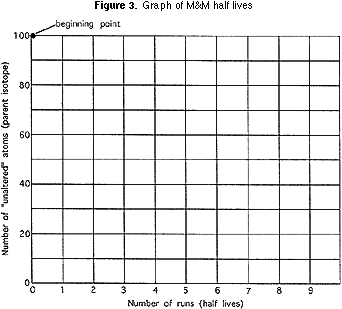Later the graphs are plotted, the instructor should guide the class into thinking near:
1) Why didn't each group become the aforementioned results?
ii) Which follows the mathematically calculated line meliorate? Is it the single group's results, or is it the line based on the class average? Why?
3) Did students have an easier time guessing (predicting) the results when in that location were a lot of pieces of candy in the cup, or when at that place were very few? Why?

U-235 is institute in well-nigh igneous rocks. Unless the stone is heated to a very loftier temperature, both the U-235 and its daughter Pb-207 remain in the rock. A geologist tin compare the proportion of U-235 atoms to Atomic number 82-207 produced from it and determine the age of the stone. The adjacent part of this do shows how this is done.
Render to top

Office 2b Activity — Each squad receives 128 apartment pieces, with U-235 written on one side and Pb-207 written on the other side. Each team is given a piece of paper marked Fourth dimension, on which is written either two, 4, 6, 8, or ten minutes.

The team should place each marked slice then that "U-235" is showing. This represents Uranium-235, which emits a serial of particles from the nucleus as it decays to Pb-207 (Pb- 207). When each team is set up with the 128 pieces all showing "U-235", a timed two-infinitesimal interval should start. During that time each team turns over half of the U-235 pieces then that they now show Pb-207. This represents one "half-life" of U-235, which is the time for half the nuclei to alter from the parent U-235 to the daughter Pb-207.

A new two-infinitesimal interval begins. During this time the squad should plough over HALF OF THE U-235 THAT WAS LEFT Later on THE Start INTERVAL OF TIME. Go on through a total of 4 to v timed intervals.

However, each team should Cease turning over pieces at the time marked on their TIME papers. That is, each team should terminate co-ordinate to their TIME paper at the end of the first timed interval (two minutes), or at the end of the 2nd timed interval (4 minutes), and so on. Subsequently all the timed intervals accept occurred, teams should exchange places with one another every bit instructed by the teacher. The task now for each team is to determine how many timed intervals (that is, how many half-lives) the set up of pieces they are looking at has experienced.

The one-half life of U-235 is 704 million years. Both the team that turned over a set of pieces and the second squad that examined the set should determine how many million years are represented past the proportion of U-235 and Pb-207 present, compare notes, and haggle virtually any differences that they got. (Right, each squad must determine the number of millions of years represented by the set that they themselves turned over, PLUS the number of millions of years represented by the set that another squad turned over.)

Office three: PUTTING DATES ON ROCKS AND FOSSILS

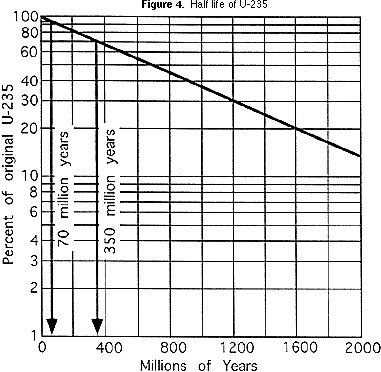
For the block diagram (Figure 1) at the beginning of this exercise, the ratio of U-235:Atomic number 82-207 atoms in the pegmatite is 1:i, and their ratio in the granite is 1:3. Using the same reasoning nearly proportions as in Part 2b above, students can determine how quondam the pegmatite and the granite are. They should write the ages of the pegmatite and granite beside the names of the rocks in the list beneath the block diagram (Figure 1).

By plotting the half life on a type of scale known equally a logarithmic scale, the curved line like that for the M & MTM activity can exist straightened out, as you tin can encounter in the graph in Figure 4. This makes the curve more than useful, considering it is easier to plot it more accurately. That is especially helpful for ratios of parent isotope to daughter isotope that represent less than one one-half life. For the block diagram (Figure 1), if a geochemical laboratory determines that the volcanic ash that is in the siltstone has a ratio of U-235:Pb-207 of 47:3 (94% of the original U-235 remains), this means that the ash is 70 1000000 years former (encounter Figure iv). If the ratio in the basalt is seven:three (seventy% of the original U-235 remains), then the basalt is 350 million years quondam (again, see Figure 4). Students should write the age of the volcanic ash abreast the shale, siltstone and basalt on the listing below the block diagram.
Return to top

QUESTIONS FOR Word

i) Based on the available radiometric ages, tin you determine the possible age of the rock unit of measurement that has acritarchs and bacteria? What is it? Why can't you say exactly what the historic period of the rock is?
2) Can y'all decide the possible age of the rock unit that has trilobites? What is it? Why can't you say exactly what the age of the rock is?
3) What is the age of the rock that contains the Triceratops fossils? Why can yous be more than precise about the age of this rock than you could nigh the ages of the rock that has the trilobites and the rock that contains acritarchs and bacteria?

Note for teachers: Based on cantankerous-cutting relationships, it was established that the pegmatite is younger than the slate and that the slate is younger than the granite. Therefore, the slate that contains the acritarch and leaner is between 704 meg years and 1408 million years sometime, considering the pegmatite is 704 meg years sometime and the granite is 1408 million years old. The slate itself cannot be radiometrically dated, and so can only be bracketed between the ages of the granite and the pegmatite.

The trilobite-bearing limestone overlies the quartz sandstone, which cross-cuts the pegmatite, and the basalt cuts through the limestone. Therefore the trilobites and the rock that contains them must be younger than 704 1000000 years (the age of the pegmatite) and older than 350 million years (the age of the basalt). The limestone itself cannot be radiometrically dated, so can only exist bracketed betwixt the ages of the granite and the pegmatite.

The Triceratops dinosaur fossils are approximately seventy million years old, because they are institute in shale and siltstone that incorporate volcanic ash radiometrically dated at 70 1000000 years. Whatsoever Triceratops found below the volcanic ash may be a fiddling older than 70 million years, and whatever plant to a higher place may be a little younger than lxx million years. The age of the Triceratops can be adamant more closely than that of the acritarchs and bacteria and that of the trilobites because the rock unit that contains the Triceratops can itself be radiometrically dated, whereas that of the other fossils could not.

---

salinaskinters.blogspot.com

Source: https://ucmp.berkeley.edu/fosrec/McKinney.html